Conic Sections: Ellipse
An ellipse is the locus of points on a plane where the sum of the distances from any point on the curve to two fixed points is constant. The two fixed points are called foci (plural of focus). An alternate definition would be that an ellipse is the path traced out by a point whose distance from a fixed point, called focus, maintains a constant ratio less than one with its distance from a straight line not passing through the focus, called the directrix.

In
an x-y
coordinate system, the ellipse with center (h,k) along with
semi-major axis, a,
and semi-minor axis b
is represented by:
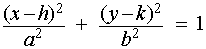
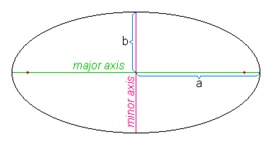
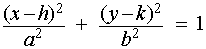

The ellipse may
be expressed parametrically by:
x = h + a cos t, -π
≤ t ≤
π
y = k + b sin t, - π ≤ t ≤ π
y = k + b sin t, - π ≤ t ≤ π
Eccentricity of Ellipse:
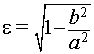
If c
equals the distance from the center to either focus, then


The distance c is known as the linear eccentricity of the ellipse.
The distance between the foci is 2aε
Area of Ellipse:
The area
enclosed by an ellipse is πab, where a and b represent the ellipse's semimajor and semiminor axes.


