Quadric Surfaces
A quadric surface is a graph of a second-degree equation in x, y, and z. Various quadric surfaces along with their equations are provided below:
Ellipsoid:
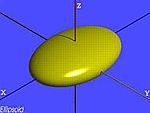

When a = b = c, the equation represents a sphere.
Elliptic Paraboloid:

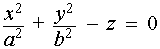
The elliptic paraboloid opens upward from its vertex residing at the origin since z ≥ 0 for all x and y. Its horizontal cross sections are ellipses for a ≠ b and circles for a = b.
Hyperbolic Paraboloid:

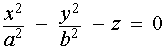
A hyperbolic paraboloid is a saddle-shaped surface. Its horizontal cross sections of are hyperbolas.
Hyperboloid of One Sheet:
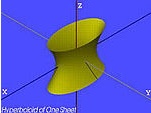
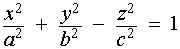
Horizontal cross sections of a hyperboloid of one sheet are ellipses for a ≠ b and circles for a = b.
Hyperboloid of Two Sheets:
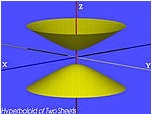
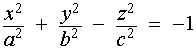
Values of z satisfying |z| > c, yield ellipses in a plane parallel to the xy plane for a ≠ b and circles in a plane parallel to the xy plane for a = b. Other values of z (i.e., z between c and −c) are not permitted since (z2/c2) − 1 ≥ 0.
Elliptical Cone:

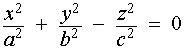
Horizontal cross sections of cones are ellipses for a ≠ b and circles for a = b except for the intersection of the cone with the plane z = 0.


