Moments About Mean
For a set of N numbers comprised of X1, X2, ..., XN, the kth moment about the mean is defined as:
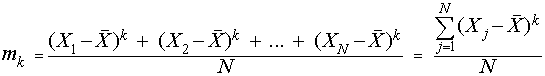
The first moment about the
mean, μ1, is zero.
The second moment about the mean, μ2, represents the variance, and is usually denoted σ2, where σ represents the standard deviation.
Find the first, second, and third moments about the mean for the set of numbers 1, 4, 6, and 9.
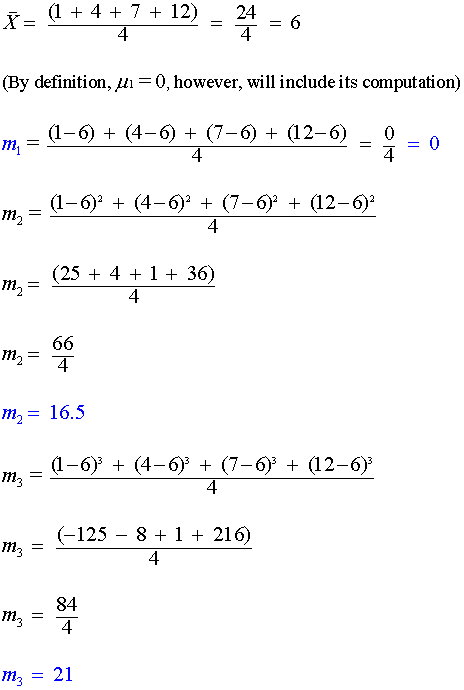
The second moment about the mean, μ2, represents the variance, and is usually denoted σ2, where σ represents the standard deviation.
Example:
Find the first, second, and third moments about the mean for the set of numbers 1, 4, 6, and 9.
Solution:
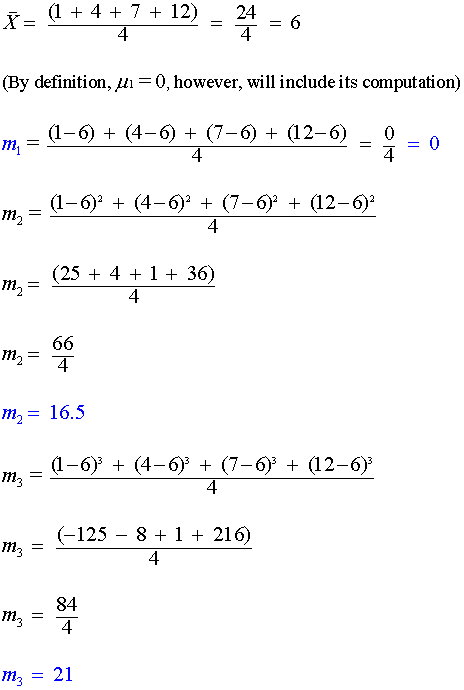
Related Topics:


