Integral
The integral of a real-valued function f of one real variable x on the interval [a, b], is denoted by
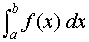
The ∫ sign, an elongated "S", represents integration; a and b are the lower limit and upper limit of integration, defining the domain of integration; f is the integrand, to be evaluated as x varies over the interval [a,b].
If a function has an integral, it is said to be integrable. The function for which the integral is calculated is called the integrand. The region over which a function is being integrated is called the domain of integration.
Properties of Integrals:

Other Topics:
- Average of an Integral
- Area Between Curves
- Volume by Cylindrical Disks
- Volume by Cylindrical Shells
- Improper Integrals


