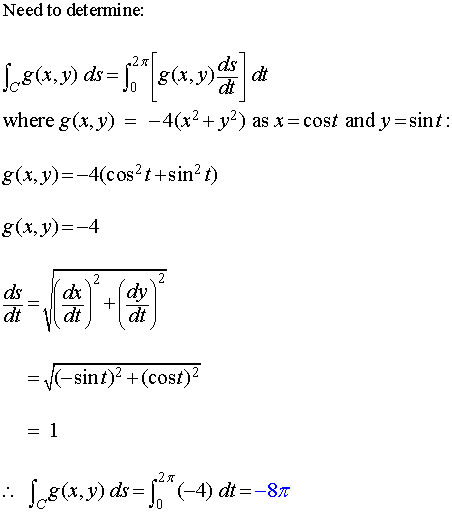Line Integral of a Scalar Field
A line integral (sometimes called a path integral) is an integral where the function to be integrated is evaluated along a curve. In the case of a closed curve it is also called a contour integral.
The function to be integrated may be a scalar field or a vector field. The value of the line integral is the sum of values of the field at all points on the curve, weighted by some scalar function on the curve (commonly arc length or, for a vector field, the scalar product of the vector field with a differential vector in the curve).
Scalar Field Definition:
Let g(x,y) be a
continuous scalar field with C:
x(t) = (x(t), y(t)), t1
≤ t
≤ t2
is a smooth curve. The line
integral of g(x,y)
along C is
defined by
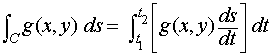
where x = x(t), y = y(t), and s is the distance measured along C.
For g(x,y) = -4(x2 + y2) with C as the unit circle: x = cos t, y = sin t for 0 ≤ t ≤ 2π , determine g(x,y).
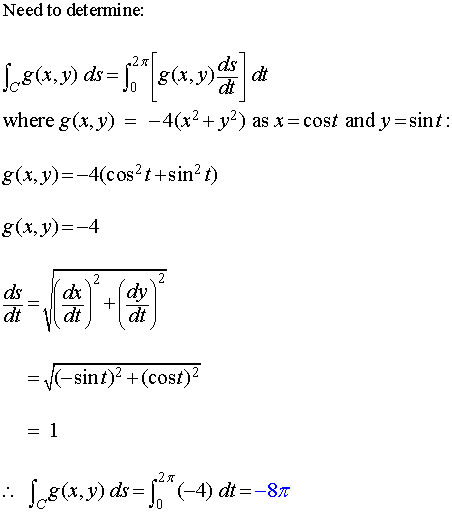
Related Topic: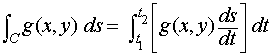
where x = x(t), y = y(t), and s is the distance measured along C.
Example:
For g(x,y) = -4(x2 + y2) with C as the unit circle: x = cos t, y = sin t for 0 ≤ t ≤ 2π , determine g(x,y).
Solution: