Exact First-Order Differential Equations
The differential equation
F(x,y) dx + G(x,y) dy = 0
is exact if
∂G/∂x
= ∂F/∂y in some simply
connected region of the plane. Solution to an exact
differential equation may be attained by determing f(x,y) where f satisfies:
∇f(x,y)
= (F(x,y),
G(x,y))
Example:
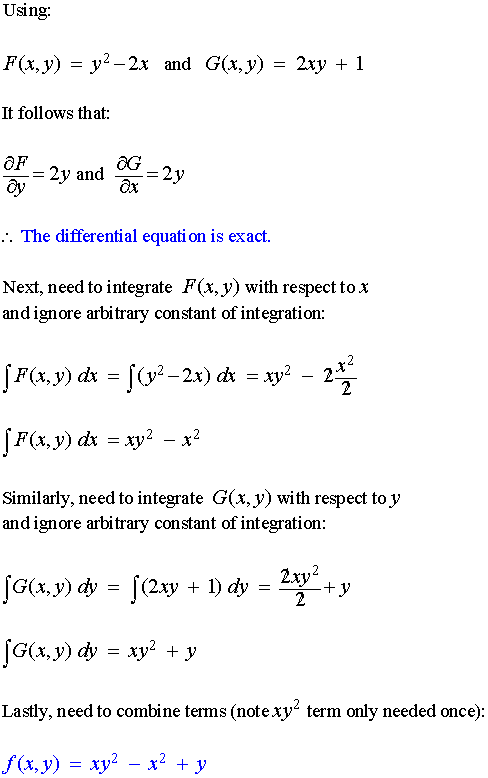
Example:
(y2 - 2x) dx + (2xy + 1) dy = 0
Determine if the above differential equation is exact. If so, solve the differential equation.
Solution:



