Isosceles Triangles

Isosceles triangles possess two sides of equal length. In addition, isosceles triangles have two congruent angles (namely, the angles opposite the congruent sides). An equilateral triangle is an isosceles triangle, but not all isosceles triangles are equilateral triangles.

Example :

For the isosceles triangle depicted below, determine the angles x and y, in addition to length from A to C.

Solution :

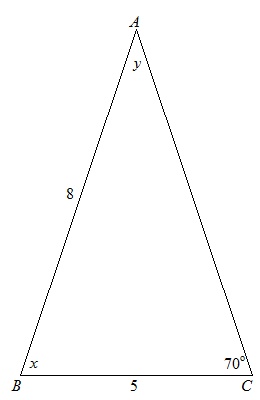
As the triangle is isosceles, it follows that AC = AB.

∴ AC = 8

As the triangle is isosceles, it follows that

m∠x = 70°

Since the sum of interior angles for a triangle equals 180°:

m∠x + 70° + m∠y = 180°

Since m∠x = 70° from above:

70° + 70° + m∠y = 180°

m∠y = 180°

-

70°

− 70°

m∠y = 40°

(Note: Inclusion of data regarding BC in the problem was merely a distractor as it was not needed to solve the problem.)