Negative Binomial Distribution
The negative binomial distribution is a discrete probability distribution, which is defined by:

where:
n = Number of trials necessary to obtain k successes
p = Probability of success for each independent trial
Unlike the binomial distribution where the number of trials is fixed and the number of successes is sought, the negative binomial distribution permits the number of successful attempts to vary while holding the number of successes at a specific value.
This is exemplified by determining the number of heads resulting from some finite number of coin tosses for the binomial distribution, whereas, the negative binomial distribution is interested in attaining the number of coin tosses necessary to achieve the desired number of heads.
The mean and variance for the negative binomial distribution are:
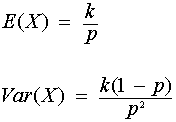
A specific location has a 10% chance of rain on any given day. What is the probability that it will take a week for it two rain on two separate days?
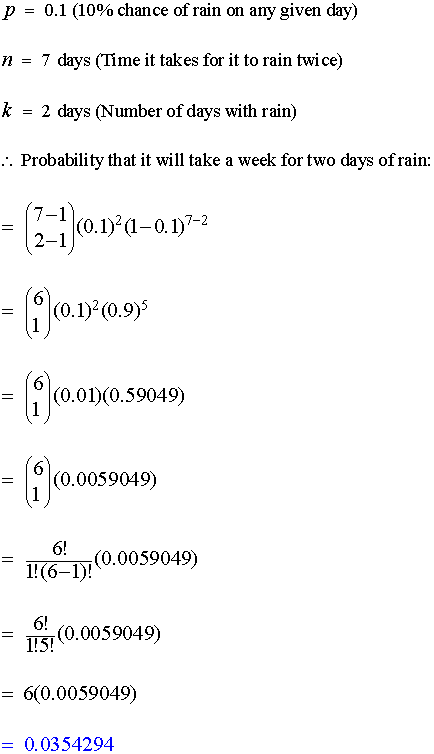
n = Number of trials necessary to obtain k successes
p = Probability of success for each independent trial
Unlike the binomial distribution where the number of trials is fixed and the number of successes is sought, the negative binomial distribution permits the number of successful attempts to vary while holding the number of successes at a specific value.
This is exemplified by determining the number of heads resulting from some finite number of coin tosses for the binomial distribution, whereas, the negative binomial distribution is interested in attaining the number of coin tosses necessary to achieve the desired number of heads.
The mean and variance for the negative binomial distribution are:
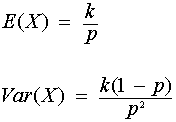
Example:
A specific location has a 10% chance of rain on any given day. What is the probability that it will take a week for it two rain on two separate days?
Solution:
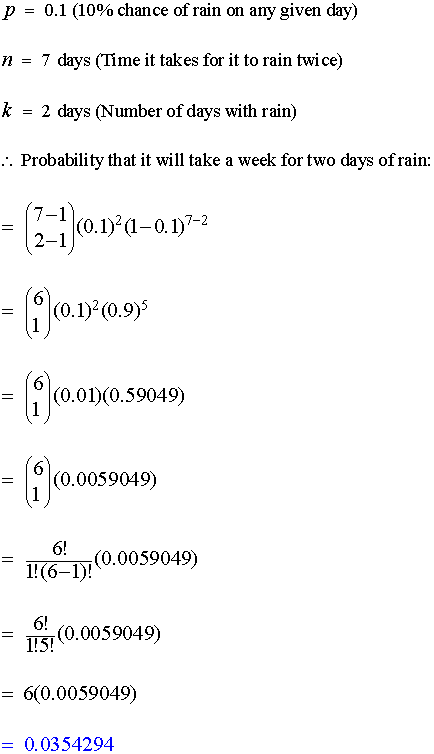
Related Topics:


